Many Body Wavefunctions
Welcome!
About this course
What can we solve in quantum mechanics?
- Particle in a box
- Harmonic oscillator H = -\frac{1}{2m}\left(\frac{d}{dx}\right)^2 +\frac{1}{2}m\omega^2 x^2
- Hydrogen atom H = -\frac{1}{2m}\nabla^2 - \frac{e^2}{r}
What would we like to solve?
Everything…
- Given ion positions \mathbf{R}_k, charge +Z_k e
H = -\frac{1}{2m}\sum_{j=1}^M\nabla^2_j + e^2\sum_{j<k}\frac{1}{|\mathbf{r}_j-\mathbf{r}_k|} - e^2\sum_{j<k}\frac{Z_k}{|\mathbf{r}_j-\mathbf{R}_k|}
Want many-electron wavefunction \Psi(\mathbf{r}_1,\ldots \mathbf{r}_N)
Hard for atoms / molecules: impossible for matter N\sim N_A!
Goal: what is a solid / metal / superconductor / superfluid?
So what do we do?
- Use simple models that we can analyse in N\to\infty limit
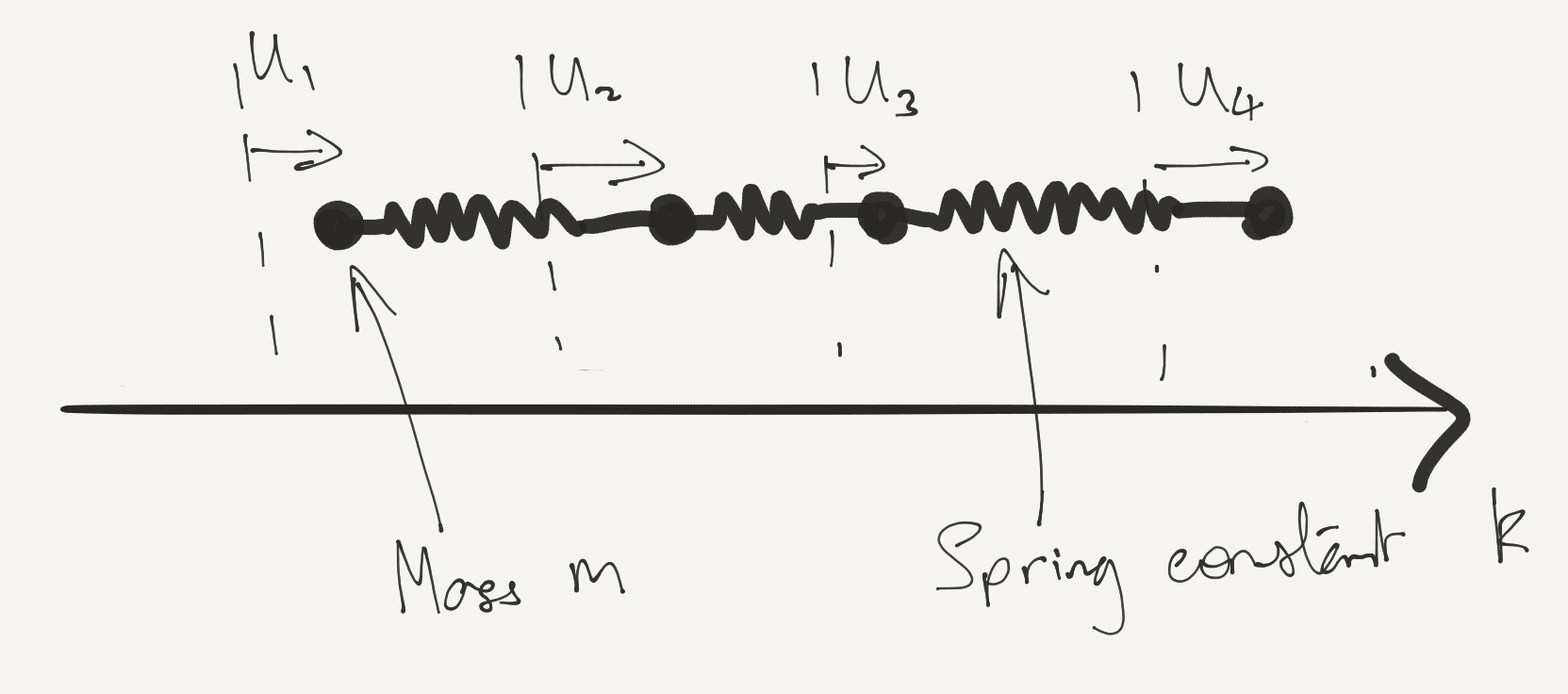
H = \sum_{j=1}^N \left[\frac{p_j^2}{2m} + \frac{k}{2} (u_j-u_{j+1})^2 \right].
- New techniques to represent wavefunctions and Hamiltonians (second quantization aka quantum field theory)
Housekeeping
Course website tqm.tripos.org (updated with slides)
Migrating from austen.uk/courses/tqm
Lecture capture (all three screens)
Lectures are 90 minutes with 5 minute break roughly halfway
Supervision details to follow
\hbar = 1!
Many Body Wavefunctions
This lecture is about the general character of many body wavefunctions and what they tell us, focusing on the simplest type: product states
Systems of indistinguishable particles are described by totally symmetric or totally antisymmetric wavefunctions
- The first kind are bosons, the second fermions
Two Particles
In general described by \Psi(\mathbf{x},\mathbf{y})
A pair of particles in states \lvert{\varphi_1}\rangle and \lvert{\varphi_2}\rangle
\Psi(\mathbf{r}_1,\mathbf{r}_2)\stackrel{?}{=} \varphi_1(\mathbf{r}_1)\varphi_2(\mathbf{r}_2)
- This would be fine for distinguishable particles
Accounting for symmetry
\Psi(\mathbf{r}_1,\mathbf{r}_2)=\frac{1}{\sqrt{2}}[\varphi_1(\mathbf{r}_1)\varphi_2(\mathbf{r}_2)\pm \varphi_2(\mathbf{r}_1)\varphi_1(\mathbf{r}_2)]
upper sign for bosons and the lower for fermions
1/\sqrt{2} for normalization if \varphi_{1,2}(\mathbf{r}) are orthonormal
When \varphi_1=\varphi_2 fermion \Psi(\mathbf{r}_1,\mathbf{r}_2) vanishes: exclusion principle
Independent Particles?
Probability density is \rho_{12}(\mathbf{r}_1,\mathbf{r}_2)=|\Psi(\mathbf{r}_1,\mathbf{r}_2)|^2
For distinguishable particles
\rho_{12}(\mathbf{r}_1,\mathbf{r}_2)=|\varphi_1(\mathbf{r}_1)\varphi_2(\mathbf{r}_2)|^2=\rho_1(\mathbf{r}_1)\rho_2(\mathbf{r}_2)
Check
Work it out for identical bosons and fermions. Show that \rho_{12}(\mathbf{r},\mathbf{r}) = 0 for fermions, and \rho_{12}(\mathbf{r},\mathbf{r}) = 2\rho_1(\mathbf{r})\rho_2(\mathbf{r}) for bosons.
- For identical particles
\begin{aligned} \rho_{12}(\mathbf{r}_1,\mathbf{r}_2) &= \frac{1}{2}\left[\rho_1(\mathbf{r}_1)\rho_2(\mathbf{r}_2)+\rho_1(\mathbf{r}_2)\rho_2(\mathbf{r}_1)\right] \\ &\pm\frac{1}{2}\left[\varphi^{}_1(\mathbf{r}_1)\varphi^*_2(\mathbf{r}_1)\varphi^{}_2(\mathbf{r}_2)\varphi^*_1(\mathbf{r}_2)+\varphi^{}_1(\mathbf{r}_2)\varphi^*_2(\mathbf{r}_2)\varphi^{}_2(\mathbf{r}_1)\varphi^*_1(\mathbf{r}_1)\right] \end{aligned}
- Identical particles in quantum mechanics are never truly independent
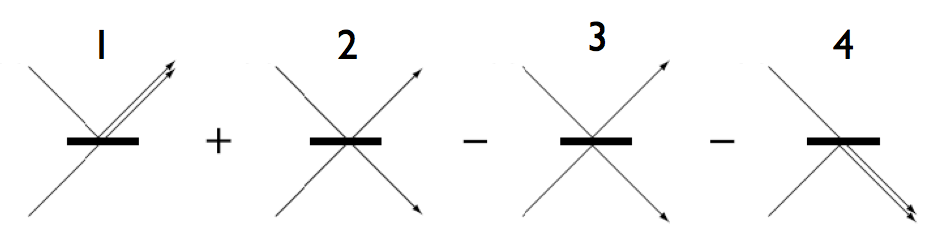
Hong–Ou–Mandel effect
Two photons (bosons) approach a beam splitter from either side
Start in orthogonal states, end up in orthogonal states e.g.
\begin{aligned} \lvert{\text{Left}}\rangle\to\frac{1}{\sqrt{2}}\left(\lvert{\text{Left}}\rangle+ \lvert{\text{Right}}\rangle\right)\\\\ \lvert{\text{Right}}\rangle\to\frac{1}{\sqrt{2}}\left(\lvert{\text{Left}}\rangle- \lvert{\text{Right}}\rangle\right) \end{aligned}
Check
If we start in \frac{1}{\sqrt{2}}[\varphi_\text{L}(\mathbf{r}_1)\varphi_\text{R}(\mathbf{r}_2)\pm \varphi_\text{R}(\mathbf{r}_1)\varphi_\text{L}(\mathbf{r}_2)] What state do we end up in?
Noninteracting particles
H = \sum_{i=1}^{N} \overbrace{\left[-\frac{\nabla_i^{2}}{2m}+V(\mathbf{r}_i)\right]}^{\equiv H_\text{sp}\text{, single particle Hamiltonian}}
H_\text{sp} has eigenstates \{\varphi_\alpha(\mathbf{r})\} and eigenenergies \{E_\alpha\}
Eigenstatate of N distinguishable particles \lvert{\Psi_{\alpha_{1}\alpha_{2}\cdots \alpha_{N}}}\rangle=\varphi_{\alpha_{1}}(\mathbf{r_{1}})\varphi_{\alpha_{2}}(\mathbf{r_{2}})\cdots\varphi_{\alpha_{N}}(\mathbf{r_{N}})
Check
Why is the energy
E = \sum_{j=1}^N E_{\alpha_j}?
Symmetrize / Antisymmetrize
\mathcal{S}=\frac{1}{N!}\sum_{\pi} P_\pi, \qquad \mathcal{A}=\frac{1}{N!}\sum_{\pi} \mathrm{sgn}(\pi)P_\pi
\pi is one of N! permutations of N objects
P_\pi is permutation operator for permutation \pi
For two particles there are two permutations: \pi=(12) and \pi=(21)
P_{(21)}\Psi(\mathbf{r}_1,\mathbf{r}_2) = \Psi(\mathbf{r}_2,\mathbf{r}_1)
Signature of Permutation
\mathrm{sgn}(\pi) is the signature of the permutation
\pm1 for \pi involving an even (odd) number of exchanges
Symmetric and antisymmetric product states
\begin{aligned} \lvert{\Psi^{S}_{\alpha_{1}\alpha_{2}\cdots\alpha_{N}}}\rangle&=\sqrt{\frac{N!}{\prod_{\alpha}N_{\alpha}!}}\mathcal{S}\,\varphi_{\alpha_{1}}(\mathbf{r_{1}})\varphi_{\alpha_{2}}(\mathbf{r_{2}})\cdots\varphi_{\alpha_{N}}(\mathbf{r_{N}}) \nonumber \\ \lvert{\Psi^{A}_{\alpha_{1}\alpha_{2}\cdots\alpha_{N}}}\rangle&=\sqrt{N!}\mathcal{A}\,\varphi_{\alpha_{1}}(\mathbf{r_{1}})\varphi_{\alpha_{2}}(\mathbf{r_{2}})\cdots\varphi_{\alpha_{N}}(\mathbf{r_{N}}) \end{aligned}
- Occupation numbers \{N_{\alpha}\} give number of particles in state \alpha
- Explicitly:
\begin{aligned} \lvert{\Psi^{S}_{\alpha_{1}\alpha_{2}\cdots\alpha_{N}}}\rangle&=\sqrt{\frac{1}{N!\prod_{\alpha}N_{\alpha}!}}\sum_\pi\varphi_{\alpha_{1}}(\mathbf{r_{\pi(1)}})\varphi_{\alpha_{2}}(\mathbf{r_{\pi(2)}})\cdots\varphi_{\alpha_{N}}(\mathbf{r_{\pi(N)}})\\ \lvert{\Psi^{A}_{\alpha_{1}\alpha_{2}\cdots\alpha_{N}}}\rangle&=\sqrt{\frac{1}{N!}}\sum_\pi \mathrm{sgn}(\pi)\varphi_{\alpha_{1}}(\mathbf{r_{\pi(1)}})\varphi_{\alpha_{2}}(\mathbf{r_{\pi(2)}})\cdots\varphi_{\alpha_{N}}(\mathbf{r_{\pi(N)}}) \end{aligned}
Check
Show that these are normalized wavefunctions if the single particle state \lvert{\varphi_\alpha}\rangle are orthonormal
Occupation Numbers
State is characterized only by occupation numbers \{N_{\alpha}\}. Use these as a label instead
Caution: To fix the sign of the fermion wavefunction, have to choose an order e.g. \varphi_{\alpha_{1}}(\mathbf{r_{1}})\varphi_{\alpha_{2}}(\mathbf{r_{2}})\cdots\varphi_{\alpha_{N}}(\mathbf{r_{N}})
The total energy is
E=\sum_{i=1}^{N}E_{\alpha_{i}}=\sum_{\alpha}N_{\alpha} E_{\alpha}
Slater Determinant
- The fermion product state is a determinant!
\lvert{\Psi^{A}_{\alpha_{1}\alpha_{2}\cdots\alpha_{N}}}\rangle=\frac{1}{\sqrt{N!}}\begin{vmatrix} \varphi_{\alpha_{1}}(\mathbf{r_{1}}) & \varphi_{\alpha_{1}}(\mathbf{r_{2}}) & \cdots & \varphi_{\alpha_{1}}(\mathbf{r_{N}}) \\ \varphi_{\alpha_{2}}(\mathbf{r_{1}}) & \cdots & \cdots & \cdots \\ \cdots & \cdots & \cdots & \cdots \\ \varphi_{\alpha_{N}}(\mathbf{r_{1}}) & \cdots & \cdots & \varphi_{\alpha_{N}}(\mathbf{r_{N}}) \end{vmatrix}
- Vanishes when two rows or two columns are identical
- Zero if two particle coordinates coincide (\mathbf{r}_{i}=\mathbf{r}_{j})
- Zero if two particles occupy the same state (\alpha_{i}=\alpha_{j})
Noninteracting particles on a ring
- Ring has circumference L. Single particle eigenstates
\varphi_{n}(x)=\frac{1}{\sqrt{L}}\exp\left(ik_n x\right)
- k_n=\frac{2\pi n}{L}, n\in\mathbb{Z}. Energies are E_{n}=\frac{k_n^2}{2m}
Ground State of Bosons
- Every particle in the state \lvert{\varphi_{0}}\rangle with zero energy: N_{0}=N
\Psi^{S}(x_{1},x_{2},\ldots x_{N})=\frac{1}{L^{N/2}}
- That was easy! The fermion case is harder
Ground State of Fermions
Fill each level with one particle, starting at the bottom (Fermi sea)
N odd: n=-(N-1)/2, -(N-3)/2,\ldots, -1, 0, 1 \ldots (N-1)/2
N even: decide whether to put the last particle at n=\pm N/2
Complex notation
z_{i}=\exp(2 \pi i x_{i}/L)
- The Slater determinant is
\Psi_0(x_1,\ldots, x_N)=\begin{vmatrix} z_{1}^{-(N-1)/2} & z_{2}^{-(N-1)/2} & \cdots & z_{N}^{-(N-1)/2} \\ z_{1}^{-(N-3)/2} & \cdots & \cdots & \cdots \\ \cdots & \cdots & \cdots & \cdots \\ z_{1}^{(N-1)/2} & \cdots & \cdots & z_{N}^{(N-1)/2} \end{vmatrix}
Simple example N=3
\begin{aligned} \Psi_0(x_1,x_2,x_3)&=\begin{vmatrix} z_{1}^{-1} & z_{2}^{-1} & z_{3}^{-1} \\ 1 & 1 & 1 \\ z_{1} & z_{2} & z_{3} \end{vmatrix} = \frac{z_{1}}{z_{2}}-\frac{z_{2}}{z_{1}}+\frac{z_{3}}{z_{1}}-\frac{z_{1}}{z_{3}}+\frac{z_{2}}{z_{3}}-\frac{z_{3}}{z_{2}}\nonumber\\ &=\left(\sqrt{\frac{z_{3}}{z_{1}}}-\sqrt{\frac{z_{1}}{z_{3}}}\right)\left(\sqrt{\frac{z_{1}}{z_{2}}}-\sqrt{\frac{z_{2}}{z_{1}}}\right)\left(\sqrt{\frac{z_{2}}{z_{3}}}-\sqrt{\frac{z_{3}}{z_{2}}}\right) \nonumber\\ &\propto \sin\left(\frac{ \pi[x_{1}-x_{2}]}{L}\right)\sin\left(\frac{ \pi[x_{3}-x_{1}]}{L}\right)\sin\left(\frac{ \pi[x_{2}-x_{3}]}{L}\right) \end{aligned}
- Wavefunction vanishes x_{i}=x_{j}
Check
Check it is periodic and totally antisymmetric
Generalization for N odd
\Psi_0(x_1,\ldots, x_N)\propto\prod_{i<j}^{N} \sin\left(\frac{\pi[x_{i}-x_{j}]}{L}\right).
Check
Show this using the Vandermonde determinant \begin{vmatrix} 1 & 1 & \cdots & 1 \\ z_{1} & z_{2} & \cdots & \cdots \\ z_{1}^{2} & z_{2}^{2} & \cdots & \cdots \\ z_{1}^{N-1} & z_{2}^{N-1} & \cdots & z_{N}^{N-1} \end{vmatrix}=\prod_{i<j}^{N}(z_{j}-z_{i})
Terminology
The wavevector of the last fermion added is called the Fermi wavevector k_\text{F}
Here k_\text{F}=\frac{(N-1)\pi}{L}
Fermi energy E_{F}=\frac{k_\text{F}^{2}}{2m} is the corresponding energy
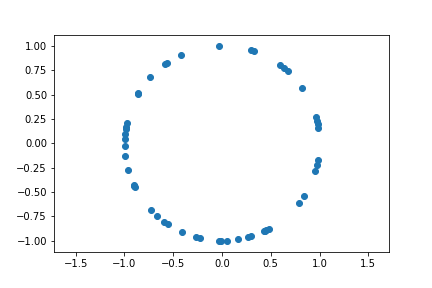
Probability distribution
- For boson ground state \Psi is constant, so is |\Psi|^2
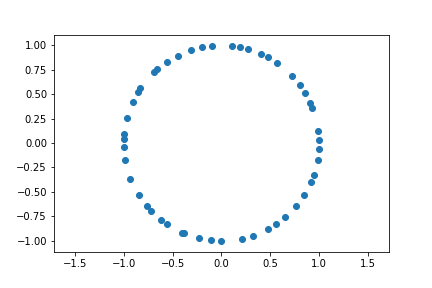
- For fermions
Marginal distributions
One particle distribution is related to average density of particles \rho_1(x_1) = N \int dx_2\ldots dx_N \lvert\Psi(x_1,x_2,\ldots,x_N)\rvert^2
Note that density is always a number density (not mass density).
Normalization of the wavefunction implies \int dx \rho_1(x) = N
In a translationally invariant system like the fermion gas on a ring we expect the average density to be constant.
Density Operator
- Regard \rho_1(x_1) as an expectation of a density operator \rho(x) = \sum_j \delta(x-x_j) so that \rho_1(x) = \langle{\Psi}\rvert \rho(x) \lvert \Psi \rangle
Single particle density matrix
g(x,y) \equiv N\int dx_2\ldots dx_N \Psi^{}(x,x_2,\ldots,x_N)\Psi^{*}(y,x_2,\ldots,x_N)
- Note g(x,x) = \rho_1(x)
Check
Starting from the Slater determinant, show that g(x,y) for the ground state of the Fermi gas is \begin{aligned} g(x,y) &= \frac{1}{L}\sum_{|k|<k_\text{F}} e^{ik(x-y)} \longrightarrow \int_{-k_\text{F}}^{k_\text{F}} \frac{dk}{2\pi} e^{ik(x-y)} \\ &\longrightarrow n \frac{\sin \left[k_\text{F}(x-y)\right]}{k_\text{F}(x-y)} \end{aligned} where n \equiv \frac{k_\text{F}}{\pi} is the average density
Pair Distribution
- Marginal probability distribution of a pair of particles \rho_2(x_1,x_2) = N(N-1) \int dx_3\ldots dx_N\left|\Psi(x_1,x_2,\ldots,x_N)\right|^2
- The prefactor is to account for all pairs of particles
Check
Starting from the Slater determinant, show that \rho_2(x_1,x_2) = n^2\left[1 - \left(\frac{\sin\left[k_\text{F}(x_1-x_2)\right]}{k_\text{F}(x_1-x_2)}\right)^2\right] This vanishes at x_1=x_2
Impenetrable Bose Gas
H = -\frac{1}{2m}\sum_j \frac{\partial^2}{\partial x_j^2} + \overbrace{c\sum_{j<k}\delta(x_j-x_k)}^{\equiv H_\text{int}}.
The second term is interaction between pairs of particles
For fermions, the interaction has no effect at all (\delta-function)!
For bosons with c\to \infty: eigenenergies coincide with those of free fermions, and eigenstates are modulus of fermion eigenstates
Check
Why?
Ground state
\Psi_0(x_1,\ldots, x_N) = \prod_{i<j}^{N} \left|\sin\left(\frac{\pi[x_{i}-x_{j}]}{L}\right)\right|.
- Can calculate any observable as long as it’s insensitive to taking the modulus (e.g. average density and pair distribution)